Temperature coefficient of resistance

3609

You might have noticed on the table for specific resistances that all figures were specified at a temperature of 20[sup]o[/sup] Celsius.  If you suspected that this meant specific resistance of a material may change with temperature, you were right!
Resistance values for conductors at any temperature other than the standard temperature (usually specified at 20 Celsius) on the specific resistance table must be determined through yet another formula:
 
The "alpha" (α) constant is known as the temperature coefficient of resistance, and symbolizes the resistance change factor per degree of temperature change.  Just as all materials have a certain specific resistance (at 20[sup]o[/sup] C), they also change resistance according to temperature by certain amounts.  For pure metals, this coefficient is a positive number, meaning that resistance increases with increasing temperature.  For the elements carbon, silicon, and germanium, this coefficient is a negative number, meaning that resistance decreases with increasing temperature.  For some metal alloys, the temperature coefficient of resistance is very close to zero, meaning that the resistance hardly changes at all with variations in temperature (a good property if you want to build a precision resistor out of metal wire!).  The following table gives the temperature coefficients of resistance for several common metals, both pure and alloy:
-----------------------------------------------------------------------------------------------------
TEMPERATURE COEFFICIENTS OF RESISTANCE, AT 20 DEGREES C

Material     Element/Alloy    "alpha" per degree Celsius
==========================================================      
Nickel -------- Element --------------- 0.005866        
Iron ---------- Element --------------- 0.005671          
Molybdenum ---- Element --------------- 0.004579        
Tungsten ------ Element --------------- 0.004403          
Aluminum ------ Element --------------- 0.004308          
Copper -------- Element --------------- 0.004041          
Silver -------- Element --------------- 0.003819          
Platinum ------ Element --------------- 0.003729          
Gold ---------- Element --------------- 0.003715          
Zinc ---------- Element --------------- 0.003847        
Steel* --------- Alloy ---------------- 0.003            
Nichrome ------- Alloy ---------------- 0.00017          
Nichrome V ----- Alloy ---------------- 0.00013            
Manganin ------- Alloy ------------ +/- 0.000015          
Constantan ----- Alloy --------------- -0.000074          
                                                                  
* = Steel alloy at 99.5 percent iron, 0.5 percent carbon
-----------------------------------------------------------------------------------------------------
Let's take a look at an example circuit to see how temperature can affect wire resistance, and consequently circuit performance:

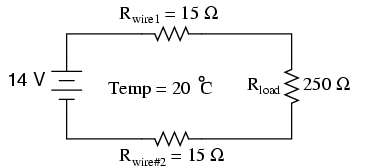
This circuit has a total wire resistance (wire 1 + wire 2) of 30 Ω at standard temperature.  Setting up a table of voltage, current, and resistance values we get:

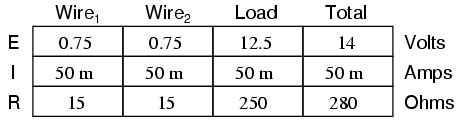
At 20[sup]o[/sup] Celsius, we get 12.5 volts across the load and a total of 1.5 volts (0.75 + 0.75) dropped across the wire resistance.  If the temperature were to rise to 35[sup]o[/sup]C Celsius, we could easily determine the change of resistance for each piece of wire. Assuming the use of copper wire (α = 0.004041) we get:

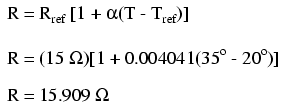
Recalculating our circuit values, we see what changes this increase in temperature will bring:
 
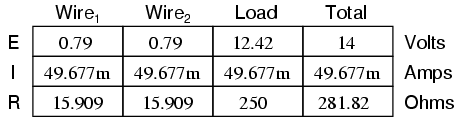
As you can see, voltage across the load went down (from 12.5 volts to12.42 volts) and voltage drop across the wires went up (from 0.75 voltsto 0.79 volts) as a result of the temperatureincreasing. Though the changes may seem small, they can be significantfor power lines stretching miles between power plants and substations,substations and loads. In fact, power utility companies often have totake line resistance changes resulting from seasonal temperature variations into account when calculating allowable system loading.

[li]REVIEW:[/li][li]Most conductive materials change specific resistance with changes in temperature.  This is why figures of specific resistance are always specified at a standard temperature (usually 20[sup]o[/sup] or 25[sup]o[/sup] Celsius).[/li][li]The resistance-change factor per degree Celsius of temperature change is called the temperature coefficient of resistance.  This factor is represented by the Greek lower-case letter "alpha" (α).[/li][li]A positive coefficient for a material means that its resistance increases with an increase in temperature.  Pure metals typically have positive temperature coefficients of resistance.  Coefficients approaching zero can be obtained by alloying certain metals.[/li][li]A negative coefficient for a material means that its resistance decreases with an increase in temperature.  Semiconductor materials (carbon, silicon, germanium) typically have negative temperature coefficients of resistance.[/li][li]The formula used to determine the resistance of a conductor at some temperature other than what is specified in a resistance table is as follows:[/li]

yjm2000

纯英文的！

轩尼诗

罚3609翻译成中文，要不就大打30大板 [s:70]  

yjm2000

3609的英文很烂的，只过了6级！

grn

lymex

补充几点
1、以前的碳膜电阻是负温度系数的，即温度增加则电阻减少
2、后来的金属膜电阻大部分是正度系数的，即温度增加则电阻也增加
3、绝大多数金属材料的温度系数为正。比如灯泡用的钨丝，常温不点燃时电阻很小，而正常点燃后电阻可能增加为10倍
4、温度系数一般用α表示，比如+0.01%/C，意味着温度每增加1度，电阻变化+0.01%
温度系数小的可以用ppm/C来表示，比如-2ppm%/C，意味着温度每增加1度，电阻减少2ppm
5、可以看出，温度既然是变化的，那么就有个参考温度点。我国是沿用苏联的标准为20度，欧美大多是23度。也就是说，平常说的电阻是多少，是指这个特定温度下的电阻Rref。这个温度，就是上面说的Tref。

6、以上论述，是基于电阻随温度是线性变化的假设的。也就是说，假如温度变化1度电阻增加0.01%，变化2度就增加0.02%，变化10度就增加0.1%。但是实际上，变化不是线性的
7、对于金属，电阻随温度的变化可以用2次曲线来表示，这更符合其真实的温度曲线
8、这样，电阻的温度就成为
R = Rref [ 1 + α(T - Tref) + β(T - Tref)^2 ]
可见，多了个2次项，其中β为2次项系数，单位是%/C2或者ppm/C2，读做每平方度ppm。
9、这样的模型说明，一个电阻，α温度系数不是固定不变的，而是温度一变化，α也跟着变化。比如对于Evanohm，β大约为-0.03ppm/C2，这说明，温度每增加1度，α将变化-0.03ppm/C。
例如对于在23度下α=-0.1ppm/C，记为α23=-0.1ppm/C，那么在24度下就有α=-0.13ppm/C即α24=-0.13ppm/C。同样有α25=-0.16ppm/C、α26=-0.19ppm/C等等。
10、这样的模型也说明，对于一个电阻，β是固定的

11、楼主贴的那些常见的金属，温度系数都很大的，比如铜的为0.004041，即0.4041%/C，因此，不少地方用铜作为温度检测，叫做铜电阻。比如SR104的温度传感器就是用铜电阻，再比如MF18里面也有一个铜电阻做补偿用
12、这些常见的金属，β温度系数都不大，由于α温度系数很大，因此温度变化时α相对变化很小就基本可以忽略了
13、但时，对于很多精密电阻，α很小，因此β就突出了。比如对于某锰铜为材料的BZ3标准电阻α23=-1ppm/C，β=-0.6ppm/C2，这个α貌似很小，但α24就变小了0.6ppm为-1.6ppm/C，α25=-2.2ppm/C，α26=-2.8ppm/C。由此可见，锰铜的电阻温度系数的变化相对很大的。
14、Evanohm的性能就比较好，一般β=-0.03，是锰铜的1/20，因此曲线弯曲很小，能在宽范围内保持温度系数很少的变化。

chq1

电阻温度系数

您可能已经注意到在桌子上的具体数字电阻，所有在指定温度20o摄氏度。如果您怀疑这意味着具体电阻材料可随温度的变化，你是正确的！
导体电阻值在任何温度以外的标准温度（通常指定为20摄氏度）的具体耐药表必须确定通过的又一公式为：

  
在“阿尔法” （ α ）不断被称为电阻温度系数，并象征着电阻变化因素每度的温度变化。正如所有的材料有一定的特定性（在20o丙类） ，他们还改变电阻根据温度的某些款项。对于纯金属，这个系数是正数，也就是说，阻力增加而增加的温度。要件碳，硅，锗，这个系数是负数，这意味着电阻随温度升高。对于一些金属合金的电阻温度系数非常接近于零，这意味着几乎没有抵抗的变化在所有的变化，温度（一个良好的知识产权如果你想建立一个高精度电阻的金属丝！ ） 。下表给出了温度系数电阻几种常见金属，纯和合金：
-------------------------------------------------- -------------------------------------------------- -
温度系数电阻，在20摄氏度

物质元素/合金“阿尔法”每摄氏度
================================================== ========
镍-------- --------------- 0.005866元
铁---------- --------------- 0.005671元
钼---- --------------- 0.004579元
钨------ --------------- 0.004403元
铝------元--------------- 0.004308
铜-------- --------------- 0.004041元
银-------- --------------- 0.003819元
白金------ --------------- 0.003729元
黄金---------- --------------- 0.003715元
锌---------- --------------- 0.003847元
钢* ---------合金---------------- 0.003
镍铬合金---------------- 0.00017 -------
镍铬合金V ----- ---------------- 0.00013
锰铜合金------- ------------ + / - 0.000015
康铜-----合金--------------- -0.000074
                                                                    
* =钢合金铁百分之99.5 ， 0.5个百分点碳
-------------------------------------------------- -------------------------------------------------- -
让我们来看看一个例子，看看赛道温度可能会影响线电阻，因此，电路性能：

  
这条赛道的总线电阻（线1 +线2 ） 30 Ω在标准温度。建立一个表的电压，电流和电阻值，我们得到：


在20o摄氏度，我们可以在12月5日伏特负荷，共有1.5伏（ 0.75 + 0.75 ）下降跨越线电阻。如果温度上升到摄氏35oC ，我们可以很容易地确定变化的抵抗每条线。假设使用铜线（ α = 0.004041 ） ，我们得到：

  
我们的电路重新计算值，我们看到什么样的变化这一温度上升将带来：

  
正如你所看到的，负载两端的电压下降（从12.5伏特to12.42伏特）和上的压降线上升（从0.75 voltsto 0.79伏特）由于temperatureincreasing 。虽然变化似乎很小，但可以significantfor电力线之间的距离延伸发电厂和变电站，变电站和负荷。事实上，电力公司通常有totake线电阻变化引起的季节性温度变化计算时考虑到允许系统负载量。

修改：
最导电材料的电阻变化的具体温度的变化。这就是为什么数字的具体阻力总是指定在标准温度（通常为20o或25o摄氏度） 。
电阻变化因素每摄氏度的温度变化称为电阻温度系数。这一因素所代表的希腊小写字母“阿尔法” （ α ） 。
一个积极的系数的物质手段，它的电阻随温度的增加。纯金属通常具有正温度系数电阻。系数接近零可以得到某些金属合金。
负系数的物质手段，它的电阻随温度的增加。半导体材料（碳，硅，锗）通常具有负温度系数电阻。
该公式用于确定导体电阻温度在一些以外什么是指明的阻力表如下：

lymex

赛道温度？哈哈，机器翻译。

jackrao

这翻译，看得我一头雾水

zy_sh_npk

文章不错难得的好文章呀。